On the Stability of Slowly Rotating Kerr Black Holes

 ^{By Amal Pushp, Affiliate Physicist at the Resonance Science Foundation}

A black hole is a solution to Einstein’s general relativistic field equations. Based on the property of angular momentum, black holes can be categorized into two types: non-rotating and rotating. The former is described by the Schwarzschild solution and the latter by the Kerr solution, originally named after its discoverers.

Perhaps all physical systems tend to move towards equilibrium irrespective of their initial conditions and similar is the case with black holes. A long-standing problem in black hole physics concerns the stability of these great astrophysical voids. The idea is to regain the original state of the black hole after the effect of an externally applied perturbation has faded away. This proves that the black hole is indeed stable. The case otherwise is an instability and would be quite a task in that it would compel us to modify Einstein’s theory.

Addressing this unsolved challenge, physicists from New Jersey’s Princeton University in a massive 900-page article claim to prove the stability of slowly rotating Kerr black holes [1]. The content of the paper is highly rigorous with regard to the mathematical standards. Although their article is yet to be peer-reviewed, one of the earlier articles upon which the researchers built their recent work has been accepted for publication [2].

The reason why the stability of black holes has been an open challenge for years is due to the fact that exact solutions of general relativity like the one found by Roy Kerr, are stationary. This means that those solutions are not physically realistic although they exist in principle. In order to evaluate the black hole’s stability, it is advisable to let it go through an applied perturbation and see whether the mathematics back engineers itself to the original solution over the period of time.

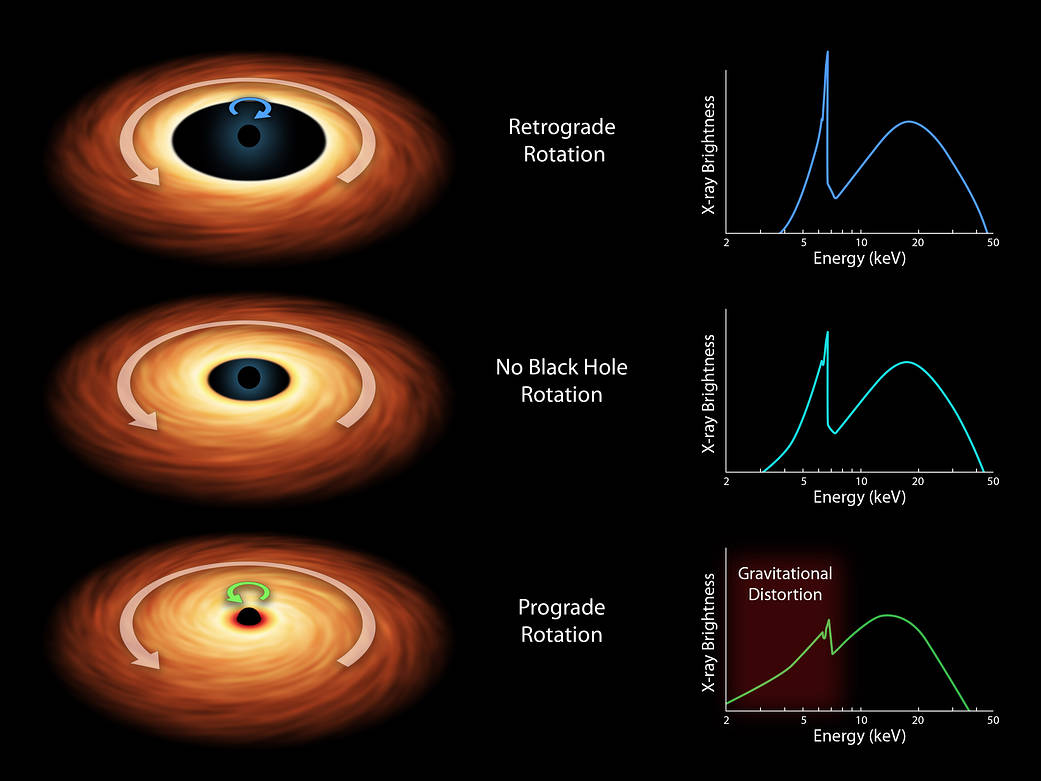^{Image credit: NASA/JPL-Caltech}

In a way, the scenario is similar to a resonance effect. The gravitational wave acts as the applied perturbation and the black hole could react in several possible ways. For an analogy consider the breaking of glass when sound waves match its resonant frequency (to learn more about resonances, please read RSF article by Dr. Inés urdaneta, entitled What is Resonance and Why is it So Important?). 

Can a similar phenomenon occur in the case of a black hole?

There are essentially four outcomes as described in the report by Quanta Magazine:

• A gravitational wave can cross the edge of a Kerr black hole and become part of its interior
• There could be significant alteration in the black hole’s mass and angular momentum, however it would still be described by the Kerr solution
• Gravity waves could revolve around the black hole before squandering in a way similar to the disappearance of sound waves encountering a glass
• Lastly, the gravitational waves might collect outside the black hole in a way that it could form an additional singularity and distort the spacetime so as to cause an instability. The Kerr solution would fail in this circumstance.

In view of the fact that there are so many possibilities, the researchers had to employ a mathematical strategy called “proof by contradiction” in order to arrive at the correct results. Basically, in this mathematical trick, the truth is established by showing that supposing a conjecture to be false leads to a contradiction. Consequently, the strategy helped the researchers conclude that Kerr black holes possessing small angular momentum are indeed stable.

RSF in Perspective:

The formation of black holes is a robust prediction of general relativity and so much progress has been made in this area since they were first theorized. Now that the stability of slowly rotating Kerr black holes has been mathematically established, it's a good head start for further developments like trying to seek proof of the final state conjecture which also depends on the Kerr stability discussed in this article.

Physicist Nassim Haramein has also made important contributions to the area of black hole physics. Interestingly, he discovered a new solution to Einstein’s general relativity by incorporating the dynamics of spin (torque and Coriolis forces) into the field equations [3]. In his model, space-time is itself curling at all the scales and this curling is the source of spin from the microscopic to the cosmological scales, reason why all black holes would rotate. His holofractographic model also suggests that the structure of a black hole is more like a torus, allowing information to collapse and expand from the central singularity. 

Given all these ideas and a lot more, a novel pursuit of the stability of black holes would be an interesting task from the telescopic view of Nassim’s theories.

References

[1] Jeremie Szeftel et al. “Wave equations estimates and the nonlinear stability of slowly rotating Kerr black holes”, https://arxiv.org/abs/2205.14808  (2022).

[2] Sergiu Klainerman, Jeremie Szeftel. “Kerr stability for small angular momentum”, https://arxiv.org/abs/2104.11857  (2021).

[3] Haramein, N., and Rauscher, E. A. (2005). The origin of spin: A consideration of torque and Coriolis forces in Einstein’s field equations and grand unification theory. Beyond The Standard Model: Searching for Unity in Physics, 1, 153-168.